Area and volume of solids of rotation

Besides finding areas below a curve definite integration can be applied to finding surface areas and volume of bodies created by rotating the curve of some fucntion about x-axis. We will calculate and show surface area and volume of one such rotational solid.

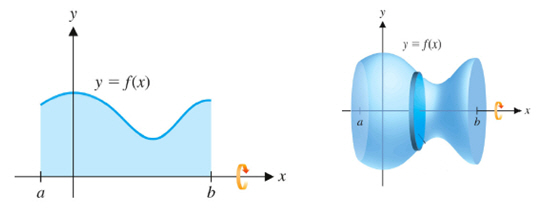
slika1 slika2

Volume of such body is given by

slika3

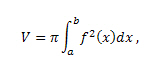
and the surface

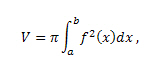
slika4

Funciton of variable x which generats rotational solid:
f(x)=
y-axis parallels a and b bounding the figure:	a =	b =

This is a Java Applet created using GeoGebra from www.geogebra.org - it looks like you don't have Java installed, please go to www.java.com

Note

It is possible to move visible part of workspace by holding SHIFT key and dragring the mousse. Zooming can be achieved by holding the SHIFT key and using the mousr scroll-wheel.

Graphics output of applet can be reset by clicking on reset icon.

Aleksandar Grujić Đajić, Created with GeoGebra